



Next: Summary
Up: The Damped Oscillator.
Previous: Over-damped Oscillations.
Contents
This corresponds to a situation where
 and the two
roots are equal. The governing equation is second order and there
still are two independent solutions. The general solution is
and the two
roots are equal. The governing equation is second order and there
still are two independent solutions. The general solution is
![\begin{displaymath}
x(t)=e^{- \beta t} [A_1 + A_2 t]
\end{displaymath}](img169.png) |
(2.20) |
The solution
![\begin{displaymath}
x(t)=x_0 e^{- \beta t} [1 + \beta t]
\end{displaymath}](img170.png) |
(2.21) |
is for an oscillator starting from rest at 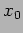 while
while
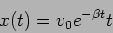 |
(2.22) |
is for a particle starting from  with speed
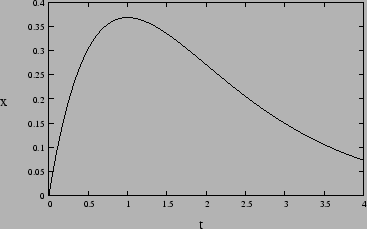
with speed 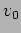 . Figure
2.3 shows the latter situation.
. Figure
2.3 shows the latter situation.
Figure 2.3:
 |
Physics 1st Year
2009-01-06